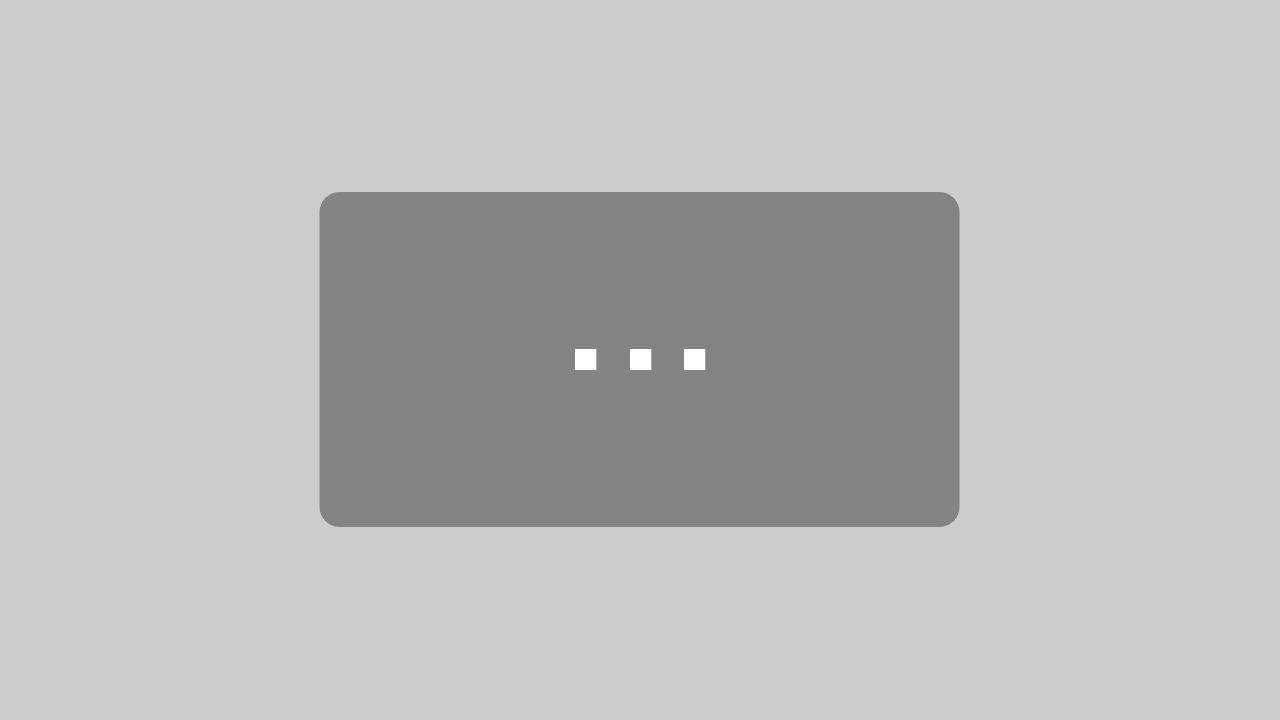
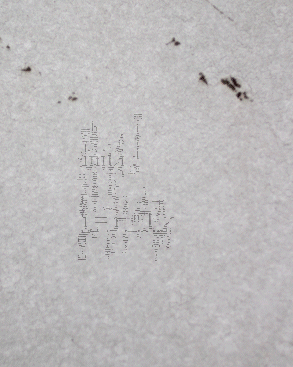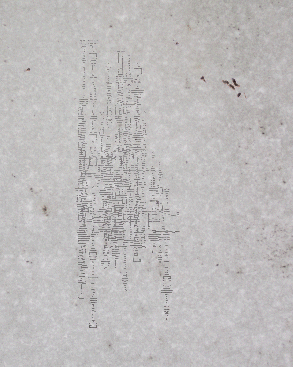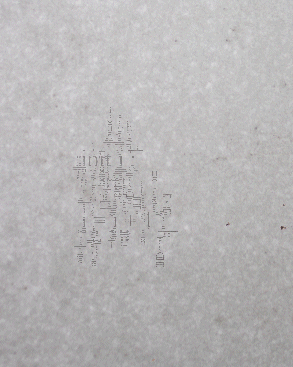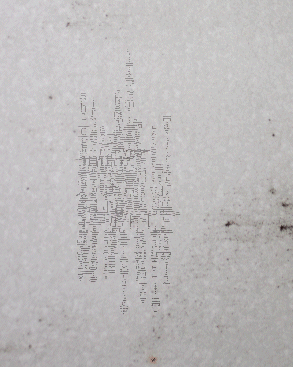
“Pixella – before the fuck”, glitch – 4th grade, 3000x2459px, 2013
Archiv der Kategorie: glitch
Dont let yourself be fooled…
I am quiet busy with my project / exhibition. Now is the time to swing the public relational hammer indeed! So be prepared to be massively advertised and properly informed about Da Product.
Get into Da Project here: http://the-code-is-cold.tk/
stay up to date with Da Blog: http://thecodeiscold.wordpress.com/blog/
Now is the time to poor in monney and support and help this WONDERFUL artwrk of MINE to live 😉
I can’t stand the rain
the code is cold | trailer #2
lisa_GLITCH
i = i + 1
“the code is cold” – manifesto
Leading up to my solo exhibition in autumn I am glad to introduce the website that will document the process of setting up the complex network of real and virtual art that is “the code is cold”.
code poetry
found:
RANKX-Funktion (DAX): RANKX(<table>, <expression>[, <value>[, <order>[, <ties>]]])
--->
Proving rank(℘(x))=rank(x)+
Enderton defines the rank of a set A to be the least ordinal α such that A⊆Vα (equivalently, A∈Vα+). He the derives the following identity: rank(A)=⋃{(rank(x))+:x∈A} for all sets A.
In Exercise 30 of Chapter 7, the reader is asked to prove several identities involving rank. For instance, that rank{a,b}=max(rank(a),rank(b))+. I am having trouble proving the second identity, that rank(℘(x))=rank(x)+ for all sets x. I am not sure whether I am missing some elementary identity that would let me prove the identity, or whether I am misunderstanding the definition of rank.
Clearly, z∈rank(℘(x))⇔(∃y)(y⊆x∧(z∈rank(y)∨z=rank(y)). On the other hand z∈(rank(x))+⇔(∃y)(y∈x∧z∈(rank(y))+)∨z=rank(x). I'm not sure why the first statement should imply the second, and conversely, however.
>> elementary-set-theory
--->
Now isn’t that poetic…
this I call home – glitch art
post privacy art #2
“before the fuck”, social glitch, 4000x3000px, 2012-2013
Sometimes, no need for glitching, society glitches all by itself. Some call this post privacy art…
“before the fuck” is an ongoing series of digital stills taken from porn sites and chat pages in that particular moment, when the user / actor leaves the room and us with that distinctive feeling of loneliness and bleak despair. It is a look inside the sadness and harshness of modern day sexuality when confronted with ‘the market’.